The work of the external and internal forces in tension (compression). Strain energy

In tension (compression) the external forces make the work in tension in consequence of displacement points where they are put (Fig. 2.10 a)

Evaluate the statically applied external force work i.e. the force which increases from zero up to its final value with a small velocity in the process of deformation.

а) б)

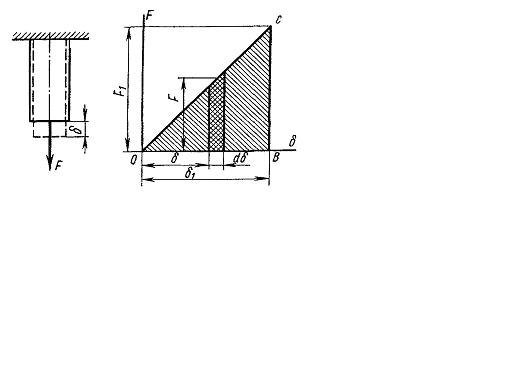
Fig. 2.10.

An elemental work dW of the external force F under the displacement 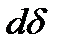is equal to

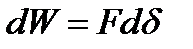. (2.22)

But there is the relation (Hook^’s law) between 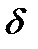and F

Substituting the value into the formula (2.22) we get

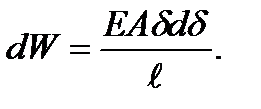
The total force work is found integrating this expression in the limits from zero to the final displacement value :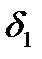

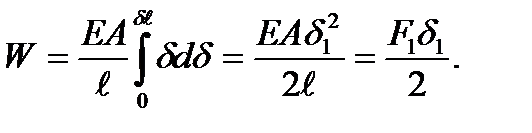
Thus,

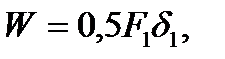(2.23)

i.e. the work of the external statically applied force is equal to half the product of the final force value and the final value of the corresponding displacement.

N

N

Fig. 2.11.

Grafically the force work F is expressed (taking into account the metric scales) by the OCB diagram area drawn in the 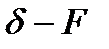coordinates (according to the scale Fig. 2.10 b).

Notice that the force work F₁ (constant by the value) under the displacement is equal to

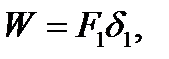(2.24)

Under the deformation both the external forces and the internal forces (elastic forces) do work.

The elemental internal force work (for the element dz) is evaluated by the formula (Fig. 2.11):

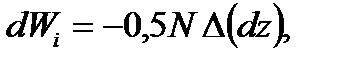(2.25)

where N is the internal force (a normal force); ∆(dz) is the element elongation.

But, according to Hook^’s law we have Hence,

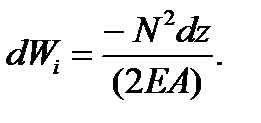(2.26)

Integrating both formula parts (2.26) we get the total internal force work for the whole bar length :

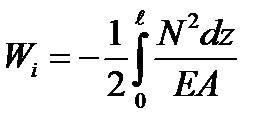. (2.27)

If N, E and A are constant, then

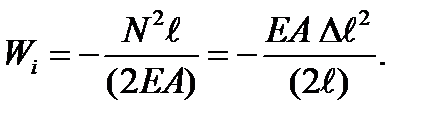(2.28)

where 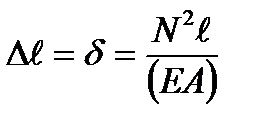is the bar elongation.

The value equal to the internal forces work but having the opposite sign is called the strain energy.

It represents the energy which is stored by the body under its deformation.

Thus, the strain energy in tension (compression) for the constant section under the action of the constant normal forces at all sections is determined by the formula

(2.29)

The strain energy set up on a unit volume of the material is called the specific potential energy:

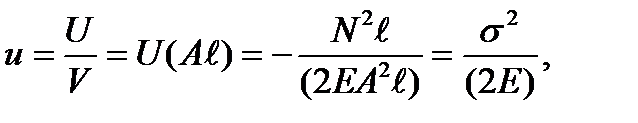(2.30)

or

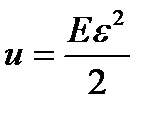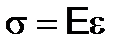as or 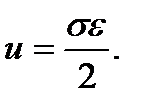(2.31)

Under the general state of stress the specific potential energy is equal to the sum of three items:

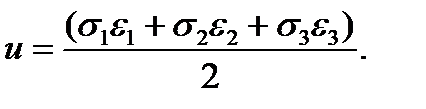(2.32)

Using the general form of Hook^’s law we get

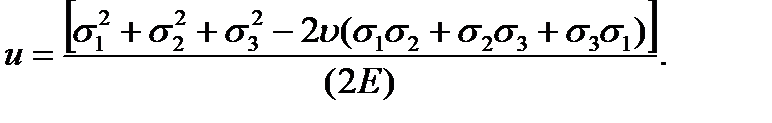(2.33)

It is easy to receive the formula of the plane stress from the formula (2.32) as the special case of one of the principal stresses being equal to zero.