Eceentrical tension (compression)

Very often the longitudinal load can be applied not to the centroid of the cross section of the bar but with some displacement (an accentricity) about the section axis (Fig. 7.4 a).

a)

b)

Zero line

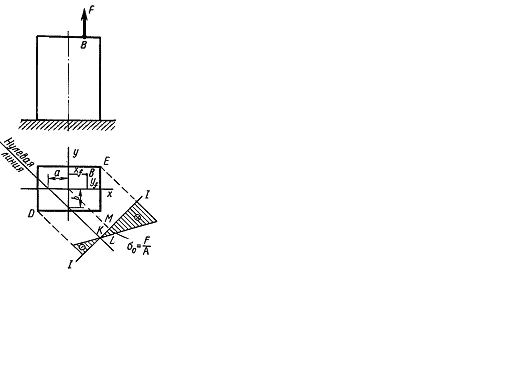
Fig. 7.4.

By using the section method we would display at any bar cross section the normal force 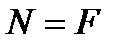and the bending moments which are equal about the x-axis 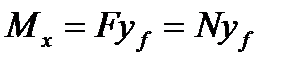and about the y-axis

Therefore the stress at any point of the cross section with the coordinates x and y are determined like that in axial tension and bending into two planes, i.e. by the formula which is analogous to the formula (7.18):

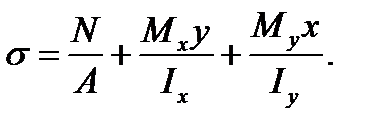(7.21)

For the sections having the overhanging angle points the stresses are determined by the formula

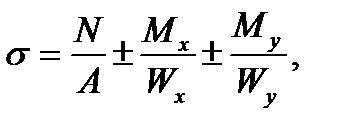(7.22)

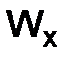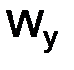where and are the section modulus about x and y-axis.

At the section shown in Fig. 7.4 b the maximum stresses will be at the point E as here the tension stresses caused by both the axial tension and bending are summed up in two planes:

(7.23)

The minimum (in algebraic sense) stresses will be at the point D:

(7.24)

Thereby they can appear both tension and compression ones.

The strength condition with the tension stresses has the form

(7.25)

If the point of the force application is on one of the principal axes of the section, for example, on the y-axis the previous formula is simplified:

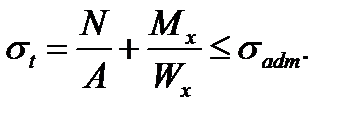(7.26)

To determine the location of the danger points for the arbitrary cross section it is necessary to find the zero line location.

By equating to zero the stress we get the zero line equation:

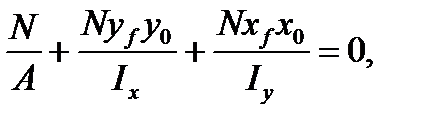(7.27)

where x₀ and y₀ are the variable coordinates of the zero line points.