Stresses at inclined sections under tension (compression) in one direction

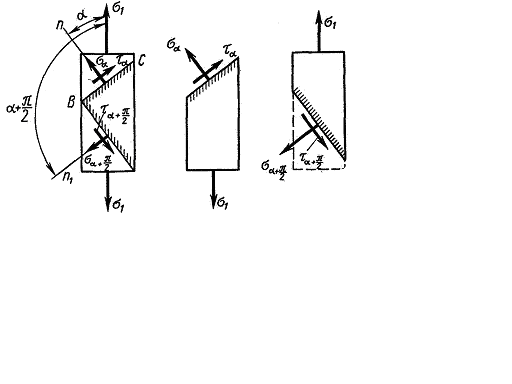
It is necessary to be able to determine the stresses acting at any inclined section of a tensed (compressed) element. To conclude about the material strength (Fig. 2.6).

а) b) c)

Fig.2.6.

Consider the normal stresses at the bar 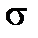cross section to be known (for example ).

Let us determine the stresses arising at the inclined BC section with the normal to it under the 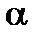angle of the 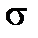direction. Accept the direction which is opposite to the clock motion as positive to count off the angle.

Denote: A is the cross-area perpendicular to the bar axis; А_α is the inclined cross area herewith

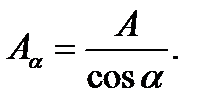(2.1)

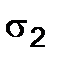normal and 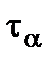shear stresses act at the inclined section in general case. We determine their values from the equilibrium condition, for example of the cut low part (Fig. 2.6 b). Project the forces on the direction:

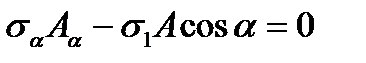. (2.2)

Using the relation (2.1) we find

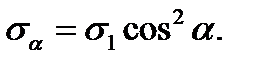(2.3)

Projecting the forces on the 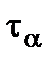direction, we get

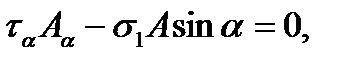
then

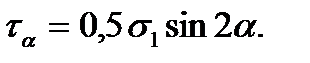(2.4)

With 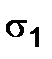value positive, (i.e. tension) and at 0 ≤ 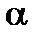≤ 90° we receive the positive value for 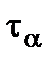. It means that the shearing stress will be as pictured in Fig. 2.6 b.

The given direction of the shearing stress is characterized by the necessity to rotate the external n normal to the section area clockwise to coincide with the shearing stress.

The shearing stresses of this direction are considered positive. If it is necessary to rotate the normal to the area against the clock to coincide with the shearing stress, this stress is then considered negative (Fig. 2.6 c).

From the formula (2.3) it follows that the maximum values of the normal stresses are set up under 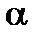=0 i.e. at the section perpendicular to the bar axis.

From the formula (2.4) it follows that under 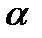= 0 the shearing stress is equal to zero.

From the formulae (2.3) and (2.4) it is evident that under 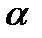= 90° we have = 0 and = 0.

Thus, the longitudinal sections have neither normal nor shearing stresses.

The areas having no shearing stresses are called principal areas and the normal stresses acting along them are the principal stresses. Consequently, the normal stress at the cross section for the tensed or compressed bar is the principal one.

It is also evident from the formula (2.4) that the maximum shearing stress takes place at the section under 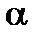= 45° and it is equal to half principal stress:

(2.5)