Figure 3.14 – Axonometry of a free circle

Figure 3.14 shows a square which is placed: a) parallel to the horizontal plane; b) – parallel to the frontal plane of projections; c) parallel to the profile plane of projections. Taken together these squares are the projection of a cube on the ortogonal planes of projections. In a rectangular dimetry this cube is shown in figure 3.4 d. It is evident, that the length of a verge to the direction of axis Y is twice less. The modeling of every point in an axonometrical projection is generally carried out after the description in figure 3.8, that is why we’ll use the well-known rule: the axonometrical projections of parallel lines are parallel between themselves. Through auxiliary points 1, 2, 3, 4, which are on axonometrical axes, draw lines parallel the proper axonometrical axes (see fig. 3.15, a) and on their crossing mark the points of ⁰B, ⁰C, ⁰E, ⁰F. Points of ⁰A, ⁰D are also found on the proper axonometrical axes. Connecting all the axonometrical projections of points, we’ll obtained an axonometrical projection of a figure (see fig. 3.15, b).

а) b)

Figure 3.15 – Axonometrical projection of a figure

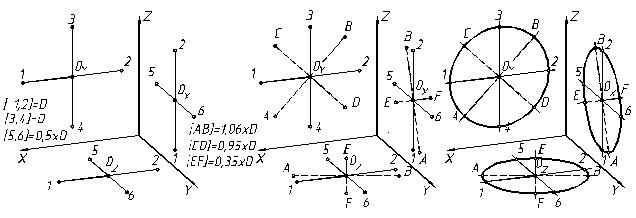
For the modeling of a rectangular dimetry circle (fig 3.16, a) which is in a co-ordinate plane (or in a plane level), first it is necessary to draw an axonometry of its center (points of 0_X, 0_Y, 0_Z (figure 3.8)), to draw through the obtained point lines, parallel to the proper two axonometrical axes (segment: [1, 2] = D; [3, 4] = D; [5, 6] = 0.5´D).

а) b) c)

Figure 3.16 – The modeling of a rectangular dimetry circle

Then we draw the minor axis of an ellipse (segment [CD] = 0,95 ´ D (dash-dotted line) or [EF] = 0,35 ´ D) placed parallell to the axonometrical axis which doesn’t exist in this plane, and also major axis (segment [AB] = 1,06 ´ D) which will be perpendicular to it (figure 3.16, b). The ellipse is traced on the eight points obtained by curve (figure 3.16, c).