Let’s consider modeling of an axonometry of point, line and plane figure in rectangular izometry.

In izometry coefficients of distortion on the axes of х, y, and z are equal and equal 1.

a) b)

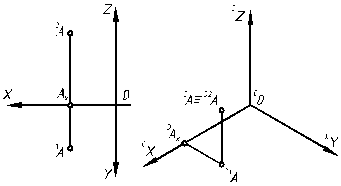
Figure 3.8 – Modeling of a point in axonometry

The segment ОА_х (figure 3.8, a) put from a point ⁰0 for the axes ⁰х of the axonometrical system of coordinates (figure 3.8, b). Through the obtained point ⁰А_х draw a straight line, parallel to⁰у, on which a segment is drown, equal a segment А_х ¹А. ^.Point ⁰¹А is obtained from which draw a straight line, parallel ^0z^.On this line draw the segment ⁰¹А⁰²А, equal the segment of А_х²А. The obtained point ⁰А is the isometric projection of point A. The axonometrical segments make an axonometrical coordinate plane.

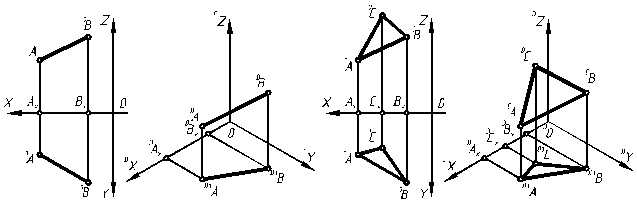
Making the considered model for every point of axonometrical figure, we can draw the model of this figure in axonometrical projections. Figure 3.9 b shows the construction of rectangular izometry for the segment AB, and figure 3.10 b shows a construction of rectangular izometry of a plane figure ABC.

а) b) а) b)

Figure 3.9 – Figure 3.10 –

Construction of rectangular izometry onstruction of rectangular izometry for the segment AB of a plane figure ABC

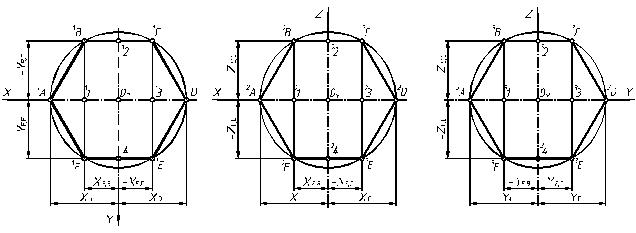
Let’s consider the modeling of rectangular izometry of a plane figurewhich is in a plane of projections (or in a level plane). As flat figures have two measurings that is why for their modeling in axonometry two axes are used, which are chosen depending on to which of the planes of projections the figure is parallel.